Archive for April, 2018

Why do we perform hypothesis tests?

       Why do we perform hypothesis tests?

A course participant commented the other day that descriptive statistical subjects were much easier to understand and could be appreciated, but not the analytical or inferential statistics which call for logical reasoning and inferential implications of the data collected.

I think the core issue lies on the abstract nature of inferential statistics. Hypothesis testing is a good example. In here, we need to determine the probability of finding the data given the truth of a stated hypothesis.

A hypothesis is a statement made that might, or might not, be true.

Usually the hypothesis is set up in such a way that it is possible for us to calculate the probability (P) of the data (or the test statistic calculated from the data) given the hypothesis, and then to make a decision about whether the hypothesis is to be accepted (high P) or rejected (low P).

A particular case of a hypothesis test is one that determines whether or not the difference between two values is significant – a significance test

For this case, we actually put forward the hypothesis that there is no real difference and the observed difference arises from random effects. We assign this as the null hypothesis (Ho).

If the probability that the data are consistent with the null hypothesis (HO) falls below a predetermined low value (say, 0.05 or 0.01), then the HO hypothesis is rejected at that probability.

Therefore, p< 005 means that if the null hypothesis were true, we would find the observed data (or more accurately, the value of the test statistic, or greater, calculated from the data) in less than 5% of repeated experiments.

To use this in significance testing, a decision about the value of the probability below which the null hypothesis is rejected, and a significance difference concluded, must be made.

In laboratory analysis, we tend to reject the null hypothesis “at the 95% level of confidence” if the probabiity of the test statistic, given the truth of HO falls below 0.05. In other words, if HO is indeed correct, less than 5% (i.e. 1 in 20 numbers) averages of repeated experiments would fall outside the limits. In this case, it is concluded that there was a significant difference.

However, it must be stressed that the figure of 95% is a somewhat arbitrary one, arising because of the fact that (mean +2 standard deviation) covers about 95% of a population.

With modern computers and spreadsheets, it is possible to calculate the probability of the statistic given a hypothesis, leaving the analyst to decide whether to accept or reject it.

In deciding what a reasonable level to accept or reject a hypothesis is, i.e. how significant is “significant”, two scenarios, in which the wrong conclusion is arrived at, need to be considered. Therefore, there is a “risk” in making a wrong decision at a specified probability.

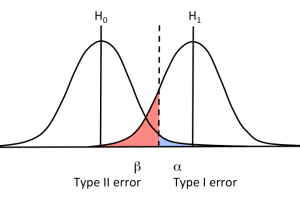
A so-called Type I error is in the case where we reject a hypothesis when it is actually true. It may also be known as “a false positive ”.

The second scenario is the opposite of this, when the significance test leads to the analyst wrongly accepting the null hypothesis although in reality HO is false (a Type II error or a false negative).

We had discuss these two types of error in the short articles: ../assets/uploads/2017/12/28/type-i-and-type-ii-errors-in-significance-tests/ ,and ../assets/uploads/2017/03/01/sharing-a-story-of-type-i-error/

Does your test result “fit for purpose”?

     The concept of “fit for purpose”

The ultimate aim of a laboratory analysis is to produce reliable enough, accurate enough results to allow the proper use of them. We do not undertake testing just for fun or for our own sake. Proper handling of the method validation and verification processes become important. And, the concept of “fit for purpose” sums up what is required.

Indeed, the quality of the analytical chemistry needs to be sufficient to answer the question on the actual situation based on sample analysis. The data user wants to know if he can eat the vegetables safely, drink the water without harm, or invest in the gold mine. Erroneous results can lead to loss of customer confidence.

In order to deliver test results that are “fit for purpose”, a proper understanding of basic statistical data analysis is essential. Unfortunately many laboratory analysts are somehow quite weak in this important subject.

To obtain valid results, we can refer to the six principles of valid analytical measurement (VAM), as proposed by the UK Laboratory of the Government Chemist (LGC):

• Analytical measurement should be made to satisfy an agreed customer requirement
• Use validated methods and equipment
• Use qualified and competent staff to undertake the task
• Participate regularly in independent assessment of technical performance (i.e. proficiency testing)
• Ensure comparability with measurement made in other laboratories (i.e. traceability, reproducibility and measurement uncertainty)
• The laboratory should have well-defined quality control and quality assurance practices.

Sampling randomization – Part II

In selecting random samples for analysis, it is necessary to generate random numbers. Random numbers also are used for simulations and can be used to create sample datasets. Random numbers can be generated in a number of different ways ……

Randomization – Part II

Sampling randomization – Part I

We have been talking about the importance of carrying out random sampling for laboratory analysis. What is actually randomization?

Randomization – Part I

Confidence intervals- How many measurements should you take?

Confidence intervals – how many measurements to take

The concept of measurement uncertainty – a new perspective

Since the publication of the newly revised ISO/IEC 17025:2017, measurement uncertainty evaluation has expanded its coverage to include sampling uncertainty as well because ISO has recognized that sampling uncertainty can be a serious factor in the final test result obtained from a given sample ……

The concept of measurement uncertainty – a new pespective