Estimation of sampling and analytical uncertainties using Excel Data Analysis toolpak
In the previous blog, we used the basic ANOVA principles to analyze the total chromium Cr data for the estimation of measurement uncertainty covering both sampling and analytical uncertainties….https://consultglp.com/2018/08/22/a-worked-example-of-measurement-uncertainty-for-a-non-homogeneous-population/
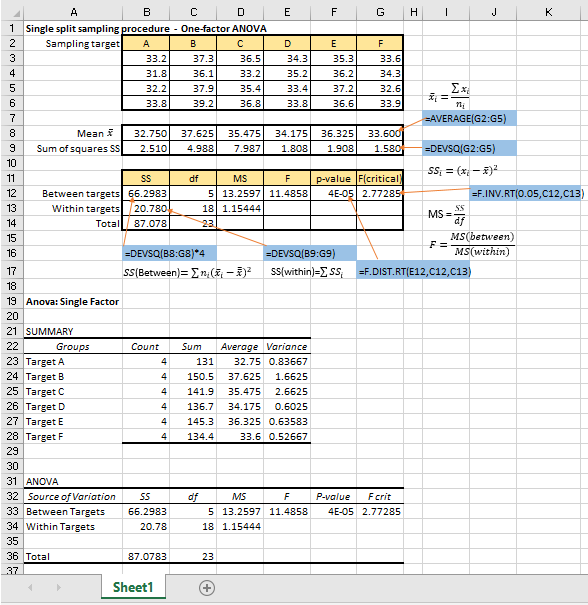
Data analysis for one factor analysis of variance ANOVA with an equal number of replicates for each ‘treatment’ is probably quicker and easier by using Excel’s traditional functions in the spreadsheet calculations.
One of the advantages is that the manual calculations return live worksheet functions rather than the static values returned by the Data Analysis Toolpak add-ins, so we can easily edit the underlying data and see immediately whether that makes sense or shows a difference to the outcome. On the other hand, we have to run the Data Analysis tool over again if we want to even change one value of the data.
The following figure shows how simple it can be in analyzing a set of test data obtained from sampling at 6 different targets of a population with the laboratory samples analyzed in four replicates. The manual calculations have been verified by the Single Factor Tool in the Data Analysis add-in, as shown in the lower half of the figure.
With the variance results in the form of MS(between) and MS(within), we can proceed easily to estimate the measurement uncertainty as contributed by the sampling and analytical components.
