All testing and calibration laboratories accredited under ISO/IEC 17025:2017 are required to prepare and implement a set of decision rules when the customer requests for a statement of conformity in the test or calibration report issued.
As the word “conformity” is defined as “compliance with standards, rules and laws”, a statement of conformity is an expression that clearly describes the state of compliance or non-compliance to a specification, standard, regulatory limits or requirements, after calibration or testing.
Like any decision made, you have to assume a certain amount of risk as you might make a wrong decision. So, how much is a risk that you can comfortably undertake when you issue a statement of conformity in your test or calibration report?
Generally, decision rules give a prescription for the acceptance or rejection of a product based on:
Certainly, you want to minimize our risk in issuing a statement of conformity that is to be proven wrong by others. But, what is the type of risk you are answering when making such decision rule? In short, it is either
From the laboratory service point of view, you should be interested in the Type I (alpha) error to protect your own interest.
Before indulging further in the discussion, let’s take note of an important assumption, that is, the uncertainty of measurement is represented by a normal (Gaussian) probability distribution function, which is consistent with the typical measurement results (being assumed the applicability of the Central Limit Theorem).
After calibration or testing an item with its measurement uncertainty known, our subsequent statement of conformance with a specification or regulatory limits can lead us to 2 possible outcomes:
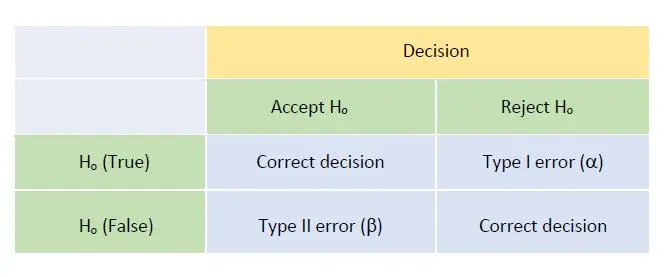
The decision rule made is related to statistical hypothesis testing where we propose a null hypothesis Ho for a situation and an alternative hypothesis H1 should Ho be rejected after some test statistics. In this case, we can make either a Type I (false POSITIVE or false ALARM, i.e. rejecting null hypothesis Ho when in fact Ho is true) or Type II (false NEGATIVE, i.e. not rejecting Ho when in fact Ho is actually false) errors.
It follows that the probabilities of making the correct decisions are (1 – alpha) and (1 – beta), respectively. Generally we would take a 5% Type I risk, hence we had alpha = 0.05 and would claim that we have 95% confidence in making this statement of conformity.
In layman’s language:
Figure 1 shows the matrix of such decision making and potential errors involved:
The statistical basis of the decision rules is to determine where the “Acceptance zone” and the “Rejection zone” are, such that if the measurement result lies in the acceptance zone, the product is declared compliant, and, if in the rejection zone, it is declared non-compliant. Graphically, it can be shown as in Figure 2 below:
Figure 2: Display of results with measurement uncertainties around specification limits
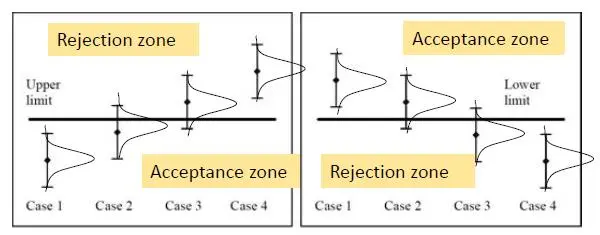
We should not have any issue in deciding the conformity in Case 1 and non-conformity in Case 4 due to a clear cut situation as shown in Figure 2 above, but we need to assess if Cases 2 and 3 are in conformity or not, as illustrated in Figure 3 below for an upper specification limit:
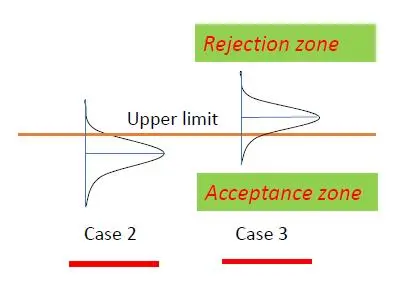
For the situations in Cases 2 and 3, we may include the following thoughts in the decision rule making before considering the amount of risk to be taken in deciding conformity:
Part B of this article will discuss both simple and more complicated decision rules that can be made during issuing statement of conformance after testing or calibration. Before that, we shall study a practical worked example.
